MatheAss 10.0 − 3-dim. Geometry

Coordinate systems

With this program, three-dimensional Cartesian coordinates can be converted into spherical coordinates or cylinder coordinates and vice versa.

cartesian            polar                           cylindrical
   x  =  1              r  =  1.7320508           ρ  =  1.4142136
   y  =  1             φ  =  45°                      φ  =  45°  
   z  =  1             Θ =  35,26439°            z  =  1

The program calculates the five Platonic bodies tetrahedron, hexahedron, octahedron, dodecahedron and icosahedron if edge length, surface height, room height, insphere radius, umbelly radius, volume or surface are given.

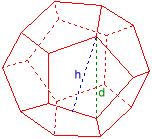
Example: Dodecahedron

Given:
¯¯¯¯¯¯
   Face Diagonal d = 2

Results:
¯¯¯¯¯¯¯
                  Edge a = 1,236068
      Face Altitude h = 1,902113
    Circumradius rc = 1,7320508
             Inradius ri = 1,3763819
              Volume V = 14,472136
             Surface S = 31,543867

Other bodies

The program calculates all the sizes of a regular prism, a vertical circular cylinder, a regular pyramid, a vertical circular cone or a sphere if two of them are given.

Example: Circular cone

Given:
¯¯¯¯¯¯
            Volume V = 1
                Base B = 1

Results:
¯¯¯¯¯¯¯
              Radius r = 0,56418958
             Altitude h = 3
           Apothem s = 3,0525907
 Lateral Surface L = 5,4105761
             Surface S = 6,4105761

Straight Line by 2 points

Line through  A(1|1|1), B(2|5|6)

Parametric representation
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  ->  ⎧ 1 ⎫     ⎧ 1 ⎫
  x = ⎪ 1 ⎪ + t·⎪ 4 ⎪
      ⎩ 1 ⎭     ⎩ 5 ⎭

Distance from origin
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  d = 0,78679579

Position to the xy plane
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
 Projection  : 4·x - y = 3
 Inters.Point: S1(0,8|0,2|0)
 Inters.Angle: 50,490288°

Position to the yz plane
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
 Projection  : 5·y - 4·z = 1
 Inters.Point: S2(0|-3|-4)
 Inters.Angle: 8,8763951°

Position to the xz plane
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
 Projection  : 5·x - z = 4
 Inters.Point: S3(0,75|0|-0,25)
 Inters.Angle: 38,112927°

Plane by 3 points

Plane through the points:
A(1|2|3), B(2|3|3), C(1|0|1)

Point-slope-form:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
->  ⎧ 1 ⎫   ⎧ 1 ⎫   ⎧ 0 ⎫
x = ⎪ 2 ⎪+r·⎪ 1 ⎪+s·⎪ 1 ⎪
    ⎩ 3 ⎭   ⎩ 0 ⎭   ⎩ 1 ⎭

Equation in coordinates:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
x - y + z = 2

Distance from origin:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
d = 1,1547005

Trace points:
¯¯¯¯¯¯¯¯¯¯¯¯¯
Sx(2|0|0) 
Sy(0|-2|0) 
Sz(0|0|2)

Sphere by 4 points

Sphere through the points: 
A(1|0|0), B(0|2|0), 
C(0|0|3), D(1|0|1)

Normal form:
¯¯¯¯¯¯¯¯¯¯¯¯
     ⎧ ->  ⎧-2,5 ⎫ ⎫² 
 K : ⎪ x - ⎪-0,5 ⎪ ⎪ = 12,75
     ⎩     ⎩ 0,5 ⎭ ⎭

Center and radius:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
 M(-2,5|-0,5|0,5)
 r = 3,5707142

Intersections in the space

The program calculates the sections of straight lines, planes and spheres.

Two Straight Lines


    ->  ⎧ 5 ⎫     ⎧ 0 ⎫
g : x = ⎪ 0 ⎪ + r·⎪ 1 ⎪
        ⎩ 0 ⎭     ⎩ 1 ⎭

    ->  ⎧ 0 ⎫     ⎧ 1 ⎫
h : x = ⎪ 5 ⎪ + s·⎪ 0 ⎪
        ⎩ 0 ⎭     ⎩ 1 ⎭

Intersection point: S(5|5|5)

Intersection angle: 60°

Distances from origin :
  d(O,g)=5  d(O,h)=5

Plane and Straight Line

Plane E :
¯¯¯¯¯¯¯¯¯
  E : x + y + z = 5

Line g :
¯¯¯¯¯¯¯¯
      ->  ⎧ 5 ⎫     ⎧ 0 ⎫
  g : x = ⎪ 0 ⎪ + r·⎪ 1 ⎪
          ⎩ 0 ⎭     ⎩ 1 ⎭

Intersection point :
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  S(5|0|0)

Intersection angle :
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  alpha = 54,73561°

Sphere and Straight Line

Sphere :
¯¯¯¯¯¯¯¯
  K : M(5|5|5) ,  r = 5

Line :
¯¯¯¯¯¯
     ->  ⎧ 1 ⎫     ⎧ 1 ⎫
 g : x = ⎪ 0 ⎪ + r·⎪ 1 ⎪
         ⎩ 0 ⎭     ⎩ 1 ⎭

Intersection points :
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
S1(2,81867|1,81867|1,81867)
S2(8,51467|7,51467|7,51467)

Length of the chord :
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  s = 9,8657657

Two Planes

Given the two planes:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  E1 : 5·x - 2·y = 5
  E2 : 2·x - y + 5·z = 8

Intersection line:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
      ->  ⎧-11 ⎫     ⎧ 10 ⎫
  g : x = ⎪-30 ⎪ + r·⎪ 25 ⎪
          ⎩  0 ⎭     ⎩  1 ⎭

Distance from origin:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  d = 1,5057283

Intersection angle:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  alpha = 65,993637°

Two Spheres

Given the two spheres:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
K1 : M1(3|3|3) ,  r1 = 3
K2 : M2(1|1|1) ,  r2 = 3

Intersection circle:
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  M(2|2|2), r = 2,4494897 

Intersection plane :
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  E : x + y + z = 6

Sphere and Plane

Plane :
¯¯¯¯¯¯¯
 E : 5·x - 4·y + 5·z = -3

Sphere :
¯¯¯¯¯¯¯¯
     ⎧ ->   ⎧ 1 ⎫⎫²
 K : ⎪ x  - ⎪ 2 ⎪⎪ = 16
     ⎩      ⎩ 3 ⎭⎭

Intersection circle :
¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯¯
  M(1|1|1),  r = 2

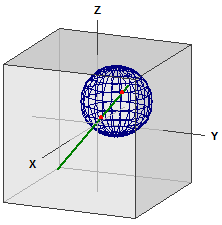
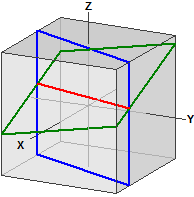
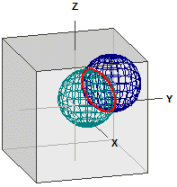
Distances on the sphere (New in version 9.0 from December 2021)

The distance between two points on a sphere is calculated. The program is also suitable for converting decimal degrees into degrees, minutes and seconds (dms) and vice versa.

GPS decimal
¯¯¯¯¯¯¯¯¯¯¯
  Berlin : 52.523403, 13.4114
New York : 40.714268, -74.005974

GPS dms
¯¯¯¯¯¯¯
  Berlin : 52° 31' 24.2508" N, 13° 24' 41.0400" E
New York : 40° 42' 51.3648" N, 74°  0' 21.5064" W
  .
  .
  .
  
Distance
¯¯¯¯¯¯¯¯
   d = r · Î± [rad] = 6385,112

eng.matheass.eu